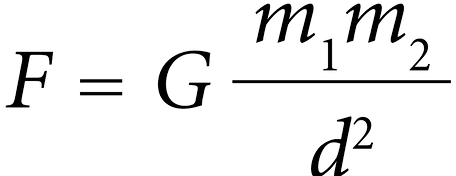Kepler's Laws are sometimes referred to as "Kepler's Empirical Laws." The reason for this is that Kepler was able to mathematically show that the positions of the planets in the sky were fit by a model that required orbits to be elliptical, the velocity of the planets in orbit to vary, and that there is a mathematical relationship between the period and the semimajor axis of the orbits. Although these were remarkable accomplishments, Kepler was unable to come up with an explanation for why his laws were true—that is, why are orbits elliptical and not circular? Why does the period of a planet determine the length of its semimajor axis?

Isaac Newton is given credit for explaining, theoretically, the answers to these questions. In his most famous work, the Principia, Newton presented his three laws:

1. An object at rest or in motion in a straight line at a constant speed will remain in that state unless acted upon by a force.

2. The acceleration of a body due to a force will be in the same direction as the force, with a magnitude indirectly proportional to its mass. (This is usually written as F = ma, or Force = mass x acceleration).

3. For every action, there is an equal and opposite reaction.

In addition, he presented his law of universal gravitation:

• The force of gravity between two masses is: F = G (m1 x m2) / d2

 

That is, the force of gravity depends on both their masses, a constant (G), and it drops off as 1 over the distance squared. In this equation, d, the distance, is measured from the center of the object. That is, if you want to know the force of gravity on you from the Earth, you should use the radius of the Earth as d, since you are that far away from the center of the Earth.

 

Using these laws and the mathematical techniques of calculus (which Newton invented), Newton was able to prove that the planets orbit the Sun because of the gravitational pull they are feeling from the Sun. The way an orbit works is as follows (this is a thought experiment attributed to Newton, sometimes called Newton's cannon):

Think of a cannon on a high mountain near the north pole of the Earth. If you were to shoot a cannonball horizontally, parallel to the Earth's surface, it would drop vertically towards the Earth's surface at the same time it is moving horizontally away from the mountain, and eventually hit the Earth. If you shot the cannonball with more force, it would travel farther from the mountain before it hit the Earth. Well, what would happen if you shot the cannonball with so much force that the amount of the vertical drop of the cannonball towards the surface due to Earth's gravity was the same magnitude as the Earth's dropoff because of its spherical shape? That is, if you could shoot a projectile with enough force, it would fall towards the Earth like any other projectile, but it would always miss hitting the Earth!

 

Although the Earth was never shot out of a cannon, the same physics applies. Think of the Earth sitting at the 3 o'clock position in its orbit around the Sun. If the Earth were to just freely fall through space without experiencing any force, by Newton's first law, it would just continue to fall in a straight line. However, the Sun is pulling on the Earth such that the Earth feels a tug towards the Sun. This causes the Earth to also fall towards the Sun a bit. The combination of the Earth falling through space and it perpetually being tugged a little bit in the direction of the Sun causes it to follow a roughly circular path around the Sun.

​

I should note here that this concept requires thinking about the concept of inertia, which can be very confusing, and, in fact, this particular animation uses terminology that may reinforce this confusion. There is no "Force of Inertia"; inertia is not a force. Instead, the proper way to think about this is that inertia is the property of an object that determines how strongly it resists changing its motion. So, picture a planet moving in a straight line; it has a lot of inertia, because a large, massive planet is hard to move off of that straight line. However, the Sun pulls on that planet with the force of gravity, and that gravitational pull is strong enough to divert the planet from a straight line path. If the tangential velocity of the planet is balanced by the change in velocity introduced by gravity, you get a stable orbit.

​

Using the techniques of calculus, you can actually derive all of Kepler's Laws from Newton's Laws. That is, you can prove that the shape of an orbit caused by the force of gravity should be an ellipse. You can show that the velocity of an object increases near perihelion and decreases near aphelion, and you can show that P2 = ka3. In fact, Newton was able to derive the value for the constant, k, and today we write Newton's version of Kepler's Third Law this way:

P2 = (4π 2 x a3) / G(m1 + m2)

Which means that k = 4π 2 / G(m1 + m2)

​

If we use Newton's version of Kepler's Third Law, we can see that if you can measure P and measure a for an object in orbit, then you can calculate the sum of the mass of the two objects! For example, in the case of the Sun and the Earth, m1 = mEarth, and m2= mSun, so just by measuring PEarth and aEarth, you can calculate mSun + mEarth!

This is the basis of a lab we are going to do during this unit. You are going to find P and a for several of Jupiter's Moons, and you are going to use those data to calculate the mass of Jupiter.

Lastly, I would like everyone to do a quick calculation using the formula for Newton's Law of Universal Gravitation:

F = G (m1 x m2) / d2

​

For now, we can ignore the constant G. We are going to calculate a ratio, so in the end the constant will drop out. What I want us to look at is the force of gravity "in space." That is, for astronauts in the space shuttle or in the International Space Station (ISS), how does the force of gravity from Earth that they feel compare to the force of gravity that you feel sitting here on Earth?

If you are unfamiliar with doing ratios, do the following step by step:

1. Write out this equation one time for the situation on Earth, that is:
   
   FonEarth = G (m1 x m2) / donEarth2

2. Write out this equation a second time for the situation in Space, that is:
   
   FinSpace = G (m1 x m2) / dinSpace2

3. Form a ratio taking the equation from #1 above and putting it over #2 above, that is:
   
   (FonEarth) / (FinSpace) = (G (m1 x m2) / donEarth2) / (G (m1 x m2) / dinSpace2)